How to destroy a Cluster of Stars/ Globular Cluster

 This blog includes - 

1.1 What are Globular clusters ?

1.2 What are Binary Stars ?

1.3 What is escape velocity ?

1.4 How to Destroy a Globular Cluster ?

1.5 Summary of Topic 

In this blog we will destroy a Cluster of Stars called Globular Cluster!

The main focus of this Blog is to make you understand how you can destroy a Globular cluster for which we will run from 1.1 to 1.3 to gather all the material to understand the topic. If you know about all of this already you can directly skip to section 1.4 to know the real tea 🍵

1.1) What are Globular clusters ? 

The name is derived by Latin word - Globulus - which means a small sphere (they are really big though- approximate size of a Globular cluster is - 300 light years) 

Globular clusters are a group of stars all formed at approximately same time and held together by gravity. ( Fig 1) They are called 'museums of stars' as they have held the stars intact since they were formed and thus help astronomers in studying age and properties of various stars( Bonus : As the stars in clusters are all formed at same time, the globular clusters help in comparing the properties of different mass stars formed at approximately same time, which is an excellent opportunity for astronomers ! ) 

fig 1

GLOBULAR CLUSTER

Although they look dense, collisions between stars in globular clusters are very rare as distances between individual stars are greater than approximate distance between stars. Moreover,  velocities of stars at any point inside the cluster are fairly random, in direction as well as size. The stars form what is called a 'collision less gas'.

Soon we will see how to boil away or destroy these museums of stars 😈

1.2) What are Binary Stars ? 

A binary system consists of two stars orbiting each other around a common centre of mass.

BINARY STARS

Given the stars in a binary system are themselves spherical, both of their orbits will be ellipses, just as for planets in the Solar System ( Bonus : for a spherical star system, the gravitational field is as such as if all of the mass is concentrated in center, when this is not the case - like when stars come close to each other tidal forces might deform the shape and resultant orbits will be complex then )

Escape velocity from a binary system : 

Let's consider a binary system of two stars orbiting around a common center of mass as shown below

Two binary stars (in purple) in orbit around a common center of mass

For simplicity, let's consider orbits to be circular and masses of both stars to be equal = M

Radius of circle = R 

In order for one of star to escape from gravitational field of other star, the kinetic energy of star should become equal the gravitational potential of the other star.

(1/2)*m*v^2 = (G*M*M)/(2*R)  (Kinetic energy = potential energy)

So, v= sqrt(GM/R)

Therefore, energy of 1 star when it has this speed

is 1/2 * m *v^2 = (G*M*M)/(2*R)   ------------ (1) 

We need to learn only this much about binary stars to understand today's topic :)

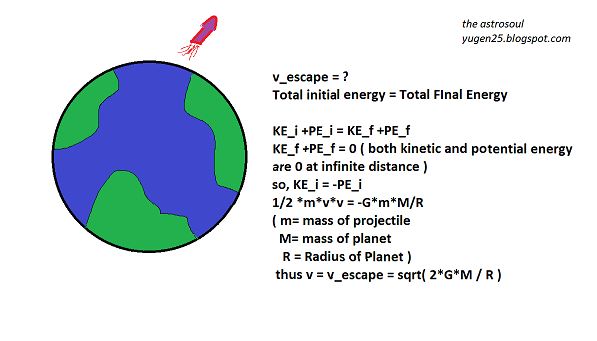
1.3) What is escape velocity ? 

The minimum velocity which is needed by a non- propelled body to escape from gravitational field of another object is called it's escape velocity. 

Formula - sqrt( 2*GM/ R ) where,  ----------(2)

G - Gravitational Constant = 6.674 x10−11 m3⋅kg−1⋅s−2

M = Mass of object which is to be escaped 

R = Radius of object which is to be escaped

Now that we are equipped with all the necessary tools to destroy a Globular cluster let's dive into it ! 

1.4) How to destroy a Globular cluster?

Let's understand what is meant by destruction of a Globular cluster. It means kicking out every star from the cluster so that it no longer remains a Globular cluster. 

Here's is the recipe, let's do it together 

Step 1. Let's calculate how much energy is needed to kick out stars from a Globular cluster?

 To do this let's take a sample cluster. 

* Mass of cluster Mcl      = Mass of 10^(6) stars, where avg. mass of 1 star = 1 Mo ( 1 solar mass ) 

* Radius of Cluster Rcl   = 50 parsecs ( pc),where 1 parsec = 3.086e+13 km 

* Escape velocity for a single star =  v_escape = sqrt( 2*G*Mcl/ Rcl ) ~ 13 km/s  ----from(2)

* Escape energy for a single star, 

   E_escape = 0.5*mass of 1 star * (v_escape)^2

                   = 2*(10^38) Joules

* Escape or destruction energy for whole cluster! 

   E_escapecluster = 

   Escape energy for a single star × total number of stars =2 × 10^38 × 10^6 

                                                                                          = 2 × 10^44 Joules

To destroy whole cluster, we need to provide 2 × 10^44 Joules energy to it. But how can we do it ?

Answer: Step 2: By forming binary pairs of stars!

Formation of a binary pair releases energy, as the two stars become bound. The energy required to form a binary pair is the same that is required to split it again. 

Thus, From (1) we know that energy released when a binary pair is formed is - (G*M*M)/(2*R)

 Suppose the stars each have one solar mass and are separated by 

the radius of a white dwarf, about 5 × 10^6 m.  Formation of such a system releases energy equivalent to 3 × 10^43 Joules.( using (1) )

Wow! only 10% of the binding energy of the cluster is provided by only one binary system. The formation of a handful of such systems could easily provide enough energy to expand the cluster or even disrupt it!!!

The energy released in this way goes to the third star by Newton's third law ( Newton's Third Law: every action has equal and opposite reaction)  and the third star now has so much energy that it simply shoots straight out of the cluster. ( This is not out of some magic, when two stars form a binary pair energy is released in a same way it is released when a particle is falling onto the star. If third star were not present, they couldn't form a binary pair ) - see the last section of this Blog ( * )to understand more accurately how the energy is actually transferred :)

Finally! We have successfully destroyed the Globular Cluster

Summary of Topic 

Globular clusters are a group of stars all formed at approximately same time and held together by gravity.

Destruction of cluster is possible by formation of a handful of binary pairs. The energy released in this way goes to the third star by Newton's third law and the third star now has so much energy that it simply shoots straight out of the cluster.

Thus one by one, shooting the stars out we can completely destroy the Globular Cluster !

___________________________________________________

                                     ( *)

(Let's take 3 stars in a Globular cluster with masses say 1, 2 and 3 solar mass. We arrange them in mercury - sun distance and give them some small initial velocity so that no star has energy to escape the three - body system. After some time, two stars become bound and the third one is expelled. 

Now how does that happen? 

The answer is in gravitational energy.When two stars become more tightly bound, they release energy, just as a particle falling onto a star releases energy . This energy goes into the motion of the third star by Newton’s third law which says that every action has equal and opposite reaction. By this law, the force exerted on the pair by the third star is exerted back on the third star by the pair: which results in binding the pair more tightly and expelling the third. If the third star were not present, the first two could not form a tight binary pair: they would fall towards one another and then recede to the same distance. )

Read More